General Relativity
Before we understand the Theory of General Relativity, let us understand some underlying concepts.
We all know this equation for energy mass conversion. That energy can be converted to mass and vice versa. But there is another great concept in this. The one that fascinated me the most. We always just notice the e and m. Let us look closely at c.
The equation said that the term c (speed of light) was a constant. In spite of all experimental data, Einstein was the first physicists to boldly state so. Let us understand the implications of this.
Say I am running at you with some speed. Now the ground I am running at you also starts moving towards you at some speed. So, my net speed has increased. Right? Right.
But if I were light, I would still be approaching you at same constant speed.
The idea that anything can have constant speed is contrary to our common-sense. We know speed and motion are relative.
But, even if the source of light was a star moving at amazing speed away from us, and another at amazing speed moving towards us or just another candle in the wind, the speed of light would always remain the same.
Another aspect. Say I am throw a stone at you faster than speed of light (well, can it travel so fast is another interesting topic), the speed of light remaining same, we will see the stone arrive after the stone actually has.
Analogous like we see the thunder first and hear it later. Only in this case, when the stone hits you, it would literally hit you before you ever saw it coming.
Establishing Relation Between Gravity and Acceleration
Let us consider two elevators: one at rest on the ground on the Earth and another, far out in space away from any gravitational pull accelerating upward with an acceleration equal to that of one Earth gravity (9.8 meters/second2).
If a ball is dropped in the elevator at rest on the Earth, it will accelerate toward the floor with an acceleration of 9.8 meters/second2. A ball released in the upward accelerating elevator far out in space will also accelerate toward the floor at 9.8 meters/second2.
Einstein used this to formulate the equivalence principle. “There is no experiment a person could conduct in a small volume of space that would distinguish between a gravitational field and an equivalent uniform acceleration.”
Hence, you can not distinguish between being weightless far out in space and being in free-fall in a gravitational field.
Suppose I am going up in an elevator somewhere in space in a space-ship at little less than speed of light. There is a hole on right side of the elevator from which a ray of light enters. As it crosses the elevator and falls on the other side, the elevator had moved up. So, I will see the ray of light bend downwards and hit the spot little lower on the other side. It may seem to be that the light is bending.
If it is going up at constant velocity, the ray of light will fall at an angle in straight line to a point lower than where it fell when the elevator was at rest.
If it is accelerating upwards, the light will appear to bend down in a curve.
Applying Equivalence Principle to the Elevator Experiment
Applying Equivalence Principle we had deduced that what seems to be effect of gravity can be seen as effect of acceleration too.
Applying it here, what seems to be effect of acceleration can be seen as effect of gravity too.
Suppose I am going up in an elevator somewhere in space in a space-ship at little less than speed of light. There is a hole on right side of the elevator from which a ray of light enters. As it crosses the elevator and falls on the other side, the elevator had moved up. So, I will see the ray of light bend downwards and hit the spot little lower on the other side. It may seem to be that the light is bending.
Now we know that earth’s gravity is acceleration. By same analogy, when I am going up on elevator, the acceleration is analogous to the gravity. So the gravity of elevator’s base is bending the light.
So by same reasoning Einstein said, a beam of light moving close to a large object like planet, will be bent by it’s gravity.
With this he changed the way we define and understand gravity. And he went ahead than that. He explained why we felt the “force” of gravity.
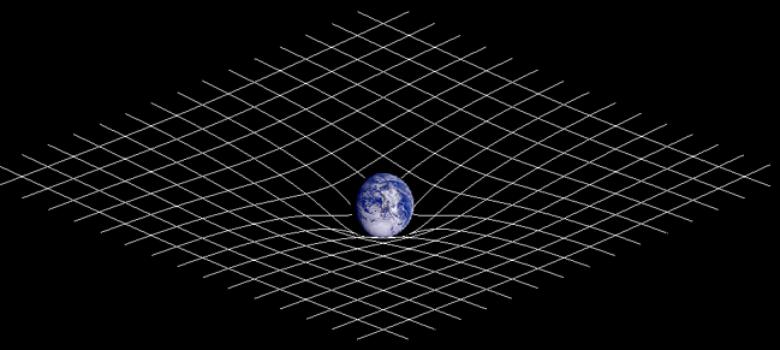
Imagine a large trampoline. Stretch it properly at a height. Put in a large ball in it and it will bend in the middle. This is how Einstein explained large bodies curve space and time. So they bend the space around them altering the geometry of the space around them. This curved space-time is what he referred to as a space-time continuum.
Now if we put a small ball on the trampoline. It will roll towards the bigger ball. That is how Einstein said, large bodies exert the “force” of gravity. Expanding on this line of thought, if you roll the small ball at an angle, it will spiral down towards the big ball. That is why bodies have orbits.
Time dilates when a body approaches the speed of light.
Say I am traveling in car that can travel nearly as the speed of light. And I have an atomic clock that is as accurate as they can be. You are standing outside the car with a clock which is in sync with my clock.
Now I travel at near speed of light for one sec by my watch. You will see me travel for more than one sec by your watch. And when we compared the clock, mine will be behind your.
It is not just time that slows down. All physical phenomenon do. Supposed we both light a cigar them burn 1 mm per second just as I start. When I stop after one second, it would have burned 1 mm. But when I stop, and we compare our cigars, yours’ would have burnt more than 1 mm.
So how much does time slow down? This is governed by the equation
T = T’ x Sqrt( 1 – (v/c)^2 )
i.e.: My time will be Time observed by you times square root of my velocity squared by speed of light squared.
Suppose, v = 0 (i.e.: my velocity is 0; I am at rest)
v/c = 0/c = 0
0^2 = 0
sqrt(0) = 0
T = T’
So as seconds are counted by our clock, they will remain in sync.
Suppose, now I travel at 1/3th the speed of light; i.e.: v = c/3;
v/c = 1/3
(1/3)^2 = 1/9
1 – 1/9 = 8/9
sqrt(8/9) = 0.942809041582063365867792482806465
Say you measured that I traveled for 1 sec (T’);
T = .9428
Thus, my atomic clock will tell me I have spent only .9428 seconds while you thought I had traveled 1 sec. The tile has slowed down for me, it has dilated!
Suppose, now I travel at the speed of light; i.e.: v = c;
v/c = 1
(1)^2 = 1
1 – 1 = 0
sqrt(0) = 0
Say you measured that I traveled for 1 sec (T’);
T = 0!!!!
Space shuttles and atomic clock have been used to test this theory and with a given range of error, the theory stands the experimental test.
Understanding Length Contraction
Similarly, the length of a body at rest (rest length) is always more than it’s length when in motion. It is governed by equation
L = L’ x sqrt( 1 – (v/c)^2 )
Where, L’ is the rest length
M = M’ / sqrt ( 1 – (v/c)^2 )
Hence, with increase in v, the mass of a body increases. A 100 kg body will have mass of 115.47 when traveling at half the speed of light.
Hendrik Antoon Lorentz was born at Arnhem, The Netherlands, on July 18, 1853. In 1878, he published an essay on the relation between the velocity of light in a medium and the density and composition thereof. The resulting formula, proposed almost simultaneously by the Danish physicist Lorenz, has become known as the Lorenz-Lorentz formula. These are the formulas I have used above to explain length contraction. His work was extended by Einstein with his paper on Theory of General and Special relativity.